The Henry George logic of wages as an economic rent

George was concerned that property owners gained all the economic rents. But his logic relies on a quite arbitrary and limited concept of property rights.

We know, for example, that human slaves could historically be the exclusive property of another person.

By George’s logic, the economic gains to human-type property owners would be an economic rent, just as the gains to location-type (or land-type) property owners are an economic rent.

From the viewpoint of property and economic rents, freeing slaves transferred a property right, in the form of the right to choose labour tasks and receive payment for them, from the slave owner to the slave.

Thus, the wages of freed slaves are the economic rent from the property right they now own. The owner of human-type property still gets the rent. It is just that each person became an own-slave-owner.

Hence, when modern Georgists say things like “all taxes come out of rents” they are just saying “all taxes come from property rights owners who can capture the economic surplus.” Or simply, “all taxes come from someone.” 

But because the understanding of property rights is limited mainly to location-type resources, not human and other resources, they miss a big part of the economic picture. While it is certainly the case that owners of location-type property need not labour for their share of economic gain, changes in the distribution of the ownership of location-type property can spread those gains more widely, just as a change in the distribution of slave-type property owners spread the gains from human labour. 

George's idea of a tax on the value of location-type property is a good way to socialise the gains from concentrated land ownership. In the modern era of high top labour incomes, taxes on the high value of these human-type property rights might also be a good way to socialise some of these economic rents.

The balance of wages, profits and rents, are not a product of physical aspects of production, but rather than property rights distributions. George somewhat indirectly makes this point when he writes:

Where land is free and labour is unassisted by capital, the whole produce will go to labour as wages.

Where land is free and labour is assisted by capital, wages will consist of the whole produce, less that part necessary to induce the storing up of labour as capital.

Where land is subject to ownership and rent arises, wages will be fixed by what labour could secure from the highest natural opportunities open to it without the payment of rent.

Where property rights to land are limited (i.e. land is free), wages and capital owners get all the surplus. As one would expect, only those with property rights can get a share of the surplus. If then property rights to capital were removed, or limited, then all the gains would go to labour. We can get any economic distribution we like with different sets of rights (aka rules). 

The relationship between George's insights on rents and the concept of property rights need more careful consideration in the modern world where the evolution of property rights tends to be invisible to many economists and policymakers. 

Downs-Thomson housing paradox

Anthony Downs and various others made the observation that “the equilibrium speed of car traffic on a road network is determined by the average door-to-door speed of equivalent journeys taken by public transport.”

Though known as the Downs-Thomson (DT) paradox, it is not a paradox at all. It is merely an observation that people adapt their behaviours to road network changes that affect the cost of driving.

The basic idea is that there are three margins from which substitution towards peak hour use of new freeway capacity occurs.

1. many drivers who formerly used alternative routes during peak hours switch to the improved expressway (spatial convergence), and
2. many drivers who formerly travelled just before or after the peak hours start travelling during those hours (time convergence), and
3. some commuters who used to take public transportation during peak hours now switch to driving, since it has become faster (modal convergence).

This is a simple application of the logic of substitution in microeconomic consumer theory. If an alternative has a lower economic cost for the same value gained, people will substitute towards the lower-cost alternative. Consumer choices are what enables markets to select for the more efficient producers and mix of goods and services over time as they respond to relative prices. 

In the DT example, the three alternatives and the peak hour freeway use start at the initial equilibrium. Prior to the new freeway, all the transport alternatives are taken up to the degree that the total cost (time/money/convenience) is equalised across them.

The cost of travelling on an alternative route, or at an alternative time, or using an alternative mode, is roughly the same as the cost of peak hour freeway transport. It must be because if it wasn’t there would be a substitution towards the lower-cost option.


If you reduce the cost of one of these alternatives what happens? That option begins to look relatively attractive, and people substitute from the other alternatives until there are no remaining gains from substitution.

Afterwards, the new equilibrium looks like this. I’ve put a dashed line at the old equilibrium to show that there can be overall gains from expanding transport capacity, even though they are not observed in the option that received the investment.[1]

How much below the previous equilibrium the cost profile of the transport alternatives remains depends on how much these costs can adjust downwards and the preference for substituting towards non-transport uses of time and money.

For example, if alternative transport modes have a fixed cost (e.g. taking the train in peak hour has a fixed price and time cost that does not change based on usage), then the new cost equilibrium will occur at the same level as previously. That fixed cost of the alternative mode will anchor the equilibrium time and cost level at which these options equilibrate.

This is what is meant by the observation that “the equilibrium speed of car traffic on a road network is determined by the average door-to-door speed of equivalent journeys taken by public transport.”[2]

Similar margins of substitution happen in housing. Housing is another spatial allocation problem where the cost involves a trade-off between price and time costs amongst alternatives.

Location alternatives are one such margin. Total cost equilibrates, via rent adjustment, between comparable dwellings at alternative locations based on relative transport/accessibility costs.

When the transport/accessibility cost of a location falls, the rent in that area rises so that there are no gains from substitution between locations.



Another margin of substitution in housing is between modes, like renting and buying. When the cost of some buying goes up, it can drag up the cost of renting as market participants close the gap between buying and renting. Alternatively, if the cost of renting falls, it tends to pull down the cost of buying.

What if there is another alternative housing mode? Say, a social or public housing option that has a fixed price. In this case, the point at which there is no substitution between them is where they all have the same economic cost.

If the cost of public housing falls, this puts pressure on the housing equilibrium. Homebuyers and renters begin substituting to this cheaper alternative, just like the case of the new freeway in transport equilibrium.

This substitution process continues until there are no gains from substituting between housing modes. Public (non-market) housing alternatives can create an anchor for prices, just as public transport alternatives anchor congestion levels.

One could argue that “the equilibrium price of housing in a private market is determined by the average price of equivalent public housing.”

Our policy choice not to extensively provide cheap housing alternatives has allowed housing prices to be anchored by the maximum willingness to pay in the market rather than the cost of public housing.

The DT paradox is why places with low traffic congestion are usually those that have good alternatives. It is also why places with cheaper private housing markets have cheaper and more widely available non-market alternatives.

[1] This is actually how monetary policy works. The overnight cash rate is manipulated, and then all the alternative interest rates shift because there is a substitution between the overnight rate and long-dated assets.

[2] The D-T paradox has implications for pricing choices on transport networks. For example, peak-hour congestion charging will raise the cost fo driving, creating substitution to alternatives, including the alternative of not travelling. When a new rail line is built to relieve pressure on roads, tickets must be priced low enough to attract people towards that alternative and away from road travel. If new rail lines are privately owned and earn a return from ticket sales only, they have an incentive to maximise their own revenue, but this may involve a ticket price that is too high to maximise overall transport gains by attracting more substitution away from road travel. New toll roads also are likely to over-price compared to the optimum for the network as a whole that would see more substitution to these new roads.

The COVID story is ideal political cover, regardless of the truth, which never mattered

NOTE: This post was written in November 2020 for a magazine that later decided it was too controversial. Some notes to reflect new information are included. 

What will I say to Mum?

When news of the first dozen COVID cases was broadcast my Mum panicked. Facts could not persuade her about the true scale of the disease. It didn’t matter what proportion of deaths COVID was responsible for, nor the chance of even more deaths from our policy reaction. It was “bad news” and “something must be done”.

So when we gather for Christmas the conversation will not drift towards this elephant in the room. The implications and outcomes of the biggest world event for decades will go unspoken when we normally reflect on the major events of the year.

COVID has introduced another tear in an already torn social fabric. No longer can we freely discuss the merits of our policy reaction, the cost of it, the human toll. You are a COVID denier or a COVID believer. There is nothing else.

I can predict that you, dear reader, are already judging me based upon the previous 140 words. That is the power of a story in human society; especially a scary one. The COVID story has changed your perception. It has changed my own conversations with my own family. The biggest effect of all—one that will be felt for years—is that this story will cover up the exorbitant exercise of political power for the benefit of political mates. It is through this mechanism that the story of COVID, not the reality of it, will cost the world dearly.

Stories and power

One of the crucial ingredients to exercising political power in favour of one’s own network is having a good cover story. One of my big mistakes in understanding the global response to COVID early on was to underestimate the power of the story. I predicted that the huge economic and health cost of locking down human society would be obvious within the first months of it occurring. I thought the story would change so that we would avoid the huge health costs of lockdowns.

What is truly scary is that the enormous costs of lockdowns are now obvious, but the lockdowns continue. Most frightening of all, most people want them!

Lockdowns are now a politically expedient response to a story that has been more infectious than COVID itself.

Partly, the use of lockdowns continues because it mostly affects the working class. The elites are insulated from this policy, at least to a degree. The main countervailing force is the small degree to which lockdowns apply to the political elite. Although politicians gain power with lockdowns and aggressive strong-man responses to COVID, like border closures, they must also personally suffer some of the consequences. That is why many elites flaunt their own rules, revealing that they are not creating and enforcing lockdown rules because they believe them to be effective, but because they understand the politics.

Harnessing the power of the scariest story yet invented

The obvious next move for the political elites is to capitalise on the virulent COVID story by transforming it into a justification for more substantial political power plays.

Normal oversight of major economic decisions is now almost non-existent, meaning favours for mates can be slipped into COVID-response policy decisions such as cash grants and subsidies to businesses who are unaffected, or rezoning and dodgy land deals. Indeed, the usual financial engineering of the central banks in response to economic shocks is mostly a giveaway in terms of insuring the risk of financial corporations.

Since first writing the above sentences the US passed the COVID stimulus bill, a monster of a document bundled together with various appropriations that seems to be written by lobbyists and contains the exact giveaways and law changes that further consolidate power.

Those who fuelled the fear should be ashamed. This was the inevitable result. Does fear ever provide the environment for the dilution of power and the sharing of wealth and prosperity? No.

2021 predictions

Note: These were first written on 21st November 2020. Some updated comments are added.

1. News media and big tech need to sell advertising, and fear sells. They will publish any story that is bad. Even vaccine news will have headlines like “The vaccine is 90% effective, but there’s a hidden catch.” Other routine medical and health data will be linked to COVID and made newsworthy to capitalise on the fear story.
2. Many useful idiots in cushy jobs desk jobs have found life to be better with COVID. Because of this, they will be subconsciously motivated to find reasons to keep the new economic order of extensive control over the lives of others. Keep an eye out for that.
3. New unrelated reasons to continue to be scared will emerge. The virus will mutate, and when the next strain arrives, the media will unleash fear-mongering headlines. Those in the game will promote it. When there are vaccine side effects, these too will be excessively reported. (Note that I wrote the previous sentences a month BEFORE the UK mutation and any vaccination approvals.)
4. Rebuilding the economy will be the cover story for huge giveaways to mates. It already has been in many ways, with untargeted cash programs, and fast-tracked planning approvals, and more. These will continue into 2021.
5. The result of this will be a rapid economic recovery. Lockdowns will mutate into sheer political theatre and behaviour policing, with limited effects on most economic production. As these lockdowns pass, macroeconomic performance will be supercharged by the huge fiscal response seen in many countries. The housing bubbles of the UK, US, Canada and Australia will continue for a few more years.
6. The battle of writing the history of 2020 will be fierce. Mainstream censorship of voices that reveal how irrational and costly the policy panic was in terms of human lives and livelihoods will continue. Sensible analysis will be shut out of social media distribution channels and even out of universities where debates and analysis are expected to take place. Technology companies have a huge incentive to police this official story because of benefits to them from the world now relying on them to an unimaginable degree. 

What are your thoughts? Just remember, the truth doesn’t matter. All major institutions will have to conform to the narrative that gets written by the powerful. As I have said before, no one in power will ever want a cost-benefit analysis of their policy choices—what is the incentive to prove yourself an idiot to the world? If anything, there will be some reports written that have obviously implausible counterfactuals and these will become the standard reference point for the official writing of the history of 2020. The story and the fear will live on through these. It will be decades before the true lessons are learnt. Such is the power of the story.

2020 was an economic lesson about insurance

My report on superannuation came out at the beginning of 2020. One of the main arguments in that report is that Australia’s superannuation system is not a retirement income system because it cannot perform an insurance function.

I didn’t know then that how we handle insurance at a society-wide level would be the most important economic policy lesson of 2020.

To explain what I mean, I must first differentiate between insurance in the financial sense and insurance in the economic sense.

Financial insurance smooths balance sheet variation when specific low-probability events occur. It works because any one person or group faces idiosyncratic risks so that pooling many people in an insurance system allows it to pay out for those few events from the funds collected from others.

But economic insurance is much different. Economic insurance deals with real resources, not financial ones.

A financial insurance system is an economic insurance system for any individual. As long as an individual’s loss of real economic resources—buildings, vehicles, equipment, stock—from an insured event is small relative to the system as a whole, these real economic losses can be easily replaced from the production of others without widespread economic disruption.

But when losses from an event are large relative to the productive economic system as a whole, simply repairing the balance sheets of individuals with cash payments will not overcome the resource loss that will affect many individuals across the system as a whole.

Consider a small island community who eat 100% of the food they grow to survive—there is not one bit of waste. If a cyclone destroys 50% of their food crops for a season, it won’t matter what sort of financial insurance systems they have in place. Their ability to feed themselves this season has declined by 50% and the loss will be felt across society, not just by the farmers whose crops were destroyed. Financial insurance cannot provide economic insurance for this type of event. The island community must suffer the loss of food and nutrition regardless of whether they can repair their financial balance sheets.

The reality of economic insurance is why I have argued in the past that a food production system that wastes a large portion of the food grown and cultivated is a type of economic insurance that provides a real resource buffer against large unforeseen shocks to the food supply system.

The military also has the features of economic insurance. Why employ tens of thousands of troops when your country is not at war? Why build and maintain ships, tanks, submarines, and jet fighters during periods they are not needed?

The answer is that financial insurance cannot stop the real resource losses from war. You must have some form of economic insurance in place with a real resource buffer being produced just in case of war. Often that requires spending years or decades devoting substantial materials and manpower to maintain a military with nothing to do.

So why is economic insurance the big lesson of 2020?

Because the health system in most countries is not run like an economic insurance system against large health shocks, even though it is clear that financial insurance will not help deal with the next pandemic. Hospitals are incentivised to run at, or near, full capacity at all times, even though health needs fluctuate, sometimes substantially, just as we have seen this year.

If we ran the health system like we do our military, or our food production systems, we would maintain a large buffer of real health resources. That would mean hospitals with surge capacity and staff maintaining them. It might involve, for example, international joint health operations to practice developing and distributing drugs and medical equipment in case of emergency.

If we ran our health system as an economic insurance system, like we do our military, that would mean an enormous expansion of health services generally. As well as being maintained as a buffer in case of emergency, these additional healthcare resources could be used for training and treating less serious health problems.

What has surprised me in 2020 is that few people are looking at the way we manage health resources and why the healthcare system is not designed as an economic insurance system like our military.

Australia's out-sized COVID stimulus

After the global financial crisis of 2008 the Australian government responded with a large fiscal stimulus.

Initially worth about $10 billion, it soon grew by $42 billion by February 2009. Out of this total about $21 billion came in the form of direct cash payments to households. 

At the same time the RBA reduced the cash rate, which flowed through a decline in variable rate mortgages from nearly 9% to around 6%. That 3% interest saving on the balance of mortgages at the time was worth around $30 billion per year. 

In total, we are looking at about $70 billion in total economic stimulus.

This scale of this stimulus effort was widely regarded to be appropriate for the circumstances of a large global macrocycle shock. 

So how big has the 2020 stimulus spending for the global COVID shock to the economy been compared to this reference point?

Early estimates of the combined fiscal and monetary stimulus were around $180 billion, or about 2.5x larger than the 2008-09 Rudd stimulus. 

On the fiscal side we have around $150 billion. 

• Initial welfare boost = $18 billion
• JobSeeker and JobKeeper and second welfare boost = $66 billion
• Business cashflow boost = $32 billion
• Early super release = $35 billion

We also have state governments increase spending and subsidies across the board, easily totalling $12 billion.

On the monetary side, we have again around $30 billion in annual interest savings to mortgage holders.  We also have the RBA intervening to lower all sorts of interest rates across the board. 

Just these items get us to nearly $200 billion, most of which came in the form of cash payments. In cash terms, rather than total value of government spending terms, the COVID stimulus measures are enormous. 

Instead of $21 billion in cash payments we are looking at nearly $130 billion in cash payments. 

Since there have been few spending options for all that extra income, the household savings rate has boomed. Households have spent $121 billion less than they earned so far this year. We can see just how much the income to households from stimulus spending has exceeded declines in incomes in the chart below. The orange bars are how much non-labour income, which includes government payments, contributed to growth in total household income. In short, disposable incomes are up massively and the main reason is the stimulus cash given to households. 

This out-sized stimulus package will have lasting effects throughout the next cycle. We have loaded up households with spending money. We have decreased the interest costs on their mortgages and encouraged them to borrow more money. We have turned off the immigration tap, meaning labour market demands can be more effectively channelled towards wage and salary growth.

To me, it seems obvious that the only thing that can happen is a massive economic surge coupled with rising asset prices. There is even a chance of a "surprise" rise in inflation. 

All of this could be seen in advance. Back in May I argued that house prices are more likely to rise than fall, and that most people had under-estimated the scale of the stimulus. 

Here's an interview were I explained my reasoning. 

The sale price of housing is not its economic price

The sale price of homes is not the economic price of housing. Prices, in the economic sense, are the relative cost of consuming newly-produced goods and services.

The economic price of housing is therefore the rental price. It represents the price of being housed today. The rental price is what goes into consumption price indexes. It goes into the national accounts to represent housing service production. It is what standard economic theory says is the price of housing.

The sale price of a house represents the purchase of a perpetual stream of housing services. In essence, you are buying the right not to have to rent a home from someone else.

Why is it important that the sale price of a house is not its economic price?

Because sale prices of housing can rise while their economic price falls. The economic price of housing translates into a sale price via other factors.

So how is the sale price of housing determined?

The asset pricing model 

The sale price of homes is determined in much the same way that the price of other rights to future income streams; the asset pricing model.

Converting the value of a flow of uncertain future income flows into a capital value today is an imperfect procedure, relying on estimates of risk, uncertainty and growth prospects. But we can for now ignore these judgements to demonstrate how the ingredients that create an asset price from the value of an income stream fit together.

Housing asset prices can be reflected by the following formula.

Asset price = (gross rent - ongoing costs) / (risk-adjusted return + property tax rate - growth expectations)

There are hence five ingredients in asset prices, of which only one is the economic price in the form of the annual rent. This is why housing asset prices can diverge so significantly from rental prices.

Let us go through each ingredient.

• Gross rent is the total amount a renter would have to pay to occupy the home for a period. It is the economic price of housing. 
• Ongoing costs include upkeep of the house—the maintenance required to sustain its current quality over time (depreciation)—as well as other ownership costs such as taxes and fees that are not levied as a fixed rate on the property value. 
• The risk-adjusted return is the rate of return buyers are will to pay for that property based on their assessment of market alternative investment returns, and the relative rate of risk of buying this house. For example, if you can get a 5% return on you money in the bank from interest, and buying this house is seen as much risker than bank deposits, then this return will be something above 5%, say 8%. 
• The property tax rate is the rate per dollar on the total property value that is required to be paid in taxes. In most US cities and states, property taxes are levied on the full asset value of the house and land, whereas in other places like Australia, these taxes are levied only on the land value component. I have adopted the US approach here for simplicity. 
• Growth expectations reflect the rate at which much buyers expect rents or prices to rise in the near future. For example, you may buy a house to avoid paying $20,000 per year rent, but rents might be rising at 5% per year. Next year, your current house purchase saves you $21,000, and it saves you $22,000 the following year. 
• We are going to simplify this model for now by pretending that growth expectations are zero. This avoids the part of the recipe that leads to big swings in the value of housing that usually self-correct over time. We will also pretend that the risk-adjusted return is best captured by the prevailing mortgage interest rate. Once you see the logic if the model, you can easily tweak these assumptions yourself to see their effect on asset prices. 

The cost of buying vs the cost of renting

Here is an example of the asset pricing model in action. A house has the following characteristics.

Rent is $20,000 per year
Ongoing costs are $5,000 per year
Mortgage rates (risk-adjusted return) are 6%
The property tax rate is 0.5%
Growth expectations are zero.

The sale price of this home will be roughly $231,000 according to the asset pricing formula [(20,000-5,000)/(0.06+0.005) = $231,000].

What this means is that you will be equally well off economically—i.e. you will spend the same each year—if you borrow the full house price amount and buy it as you would be renting it. We can add up the annual costs of buying to seeing that it is the same as renting.

Interest = $13,850 ($231,000 x 0.06)
Ongoing costs = $5,000
Taxes = $1,150 ($231,000 x 0.005)
Total = $20,000

You might notice that I have only considered the interest cost of a mortgage, not the total repayment. But paying the principle of the house is not an economic cost. It is an asset investment just like paying listed equities is an investment, not an economic cost.

Our homebuyer in this situation is paying a $20,000 economic cost of housing. But if they pay the principle of their loan over 30 years, the mortgage repayment is $16,780 per year, or $2,930 more than the interest alone. Their out-of-pocket expenses are $22,930, but that include the purchase of $2,930 of housing equity each year.

The effect of low interest rates 

Most of the change in housing sale prices over the past decade are not due to the economic price of housing as the chart below shows. Rents have been flat in Australia, and in many cities globally, while sale prices have grown enormously. 

If recent sale price changes are not due to rents, then they must be due to one of the other ingredients in the asset pricing model.

We can eliminate the ongoing costs of upkeep. Construction costs have been steady, and fixed fees and charges also have seen little variation. Property tax rates are also relatively unchanged, at least in Australia. Note also that higher property taxes reduce asset prices.

We are now down to two factors—the risk-adjusted return and growth expectations.

It could be that buyers of housing have increased their expectations of rental growth. Though after a decade of flat or falling rents, rental growth expectations should certainly have been curtailed, rather than increased.

This leaves only the interest rate. To me, this is the big story for housing prices over the past two decades globally.

We can take our previous example dwelling where we calculated its value to be $231,000 when mortgage interest rates were 6%. Now, mortgage interest rates are closer to 2%. At what price does buying cost the same as renting at this much lower rate?

Again assuming zero growth expectations, our asset pricing formula is (20,000 - 5,000) / (0.02 + 0.005) = $600,000. That’s a 160% increase in sale price, while the economic price of house remains unchanged.

We can check this calculation to ensure that the economic cost of buying at this price remains the same as before.

Interest = $12,000 ($600,000 x 0.02)
Ongoing costs = $5,000
Taxes = $3,000 ($600,000 x 0.005)
Total = $20,000

Notice that the interest paid on a mortgage is actually lower in this case, though the annual taxes are higher due to the higher sale price.

What about property taxes? 

At lower interest rates the effect of property taxes on asset prices increases. Let us compare the sale price of our example house in two different jurisdictions—one with a property tax rate of 0.5%, and one with a property tax rate of 2%.

First, we can see the effect of property taxes in our high 6% interest rate scenario. Recall that the asset price with a 0.5% property tax rate was $231,000. If we now put a 2% property tax rate in our asset pricing equation, the house is worth $187,500. Compared to this high-tax region, the asset price of the same house in a low-tax region will be 23% higher. That is a huge difference. Yet the economic price of housing is the same.

In the low interest rate scenario our house was worth $600,000 in the 0.5% low property tax area. If we plug in a 2% property tax rate and a 2% interest rate we get a value of $375,000 in the high-tax region. The sale price is now 60% higher in the low tax area for the same economic price of housing. The low interest rate environment amplifies the price effect of different property tax rates.

We can see the components of the economic price in the high property tax area in the low interest rate scenario below. 

 
Interest = $7,500 ($375,000 x 0.02)

Ongoing costs = $5,000
Taxes = $7,500($375,000 x 0.02)
Total = $20,000

We can therefore use the asset pricing model to predict that the recently-announced reductions in property tax rates in Harris County (Houston) will fuel price growth in the current low interest rate environment. We can also predict that states like Texas, with comparably high property tax rates (often 2% and above) will have even greater housing asset price divergence compared to regions with low (sub 1%) property tax rates.

Predicting strange price differences

Strange house price differences make more sense through the lens of asset pricing.

Here is an example of the types of strange price differences commonly noted. Here are two houses where the asset price seems unrelated to the quality or size of the dwelling.

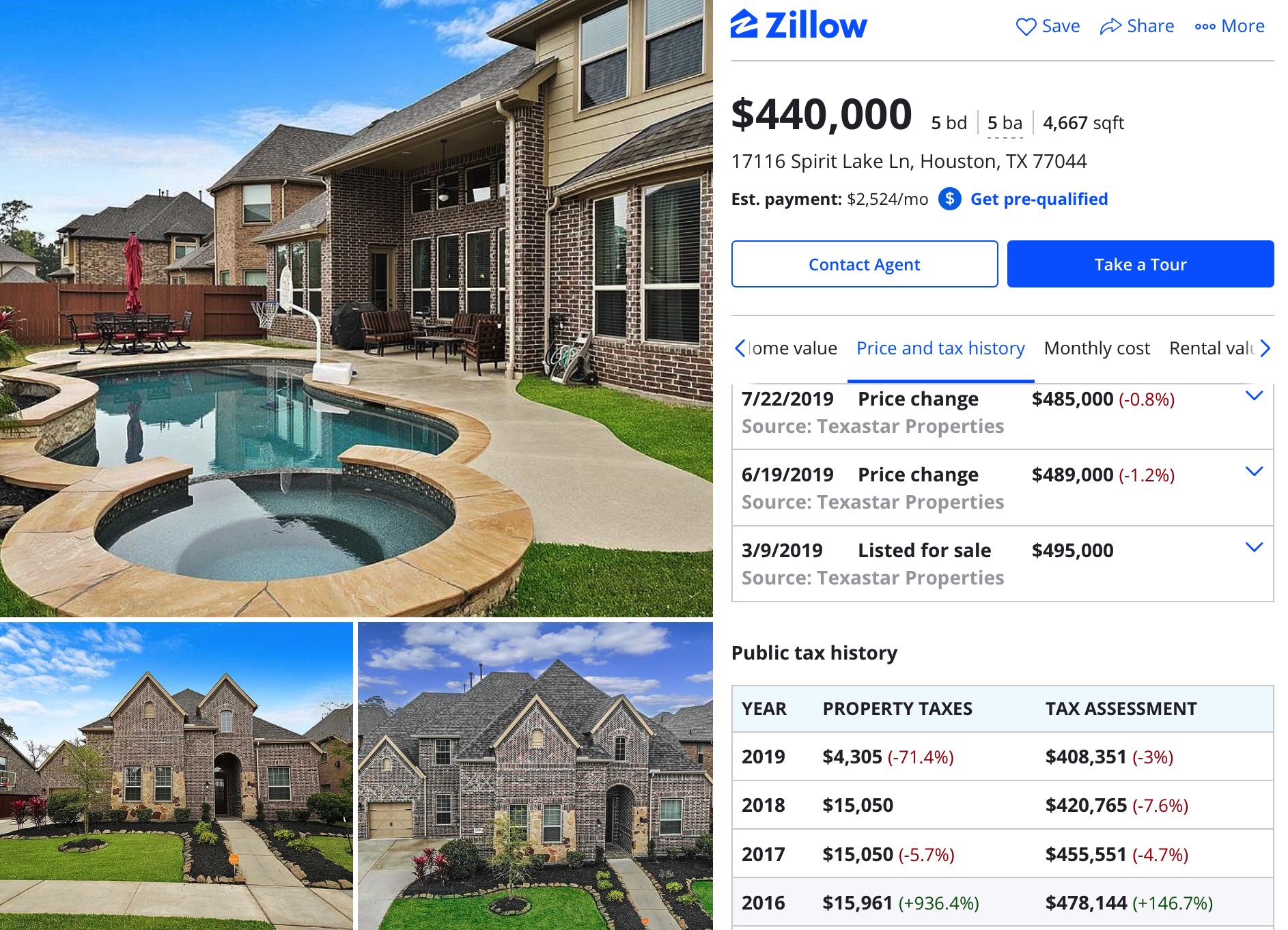
House 1

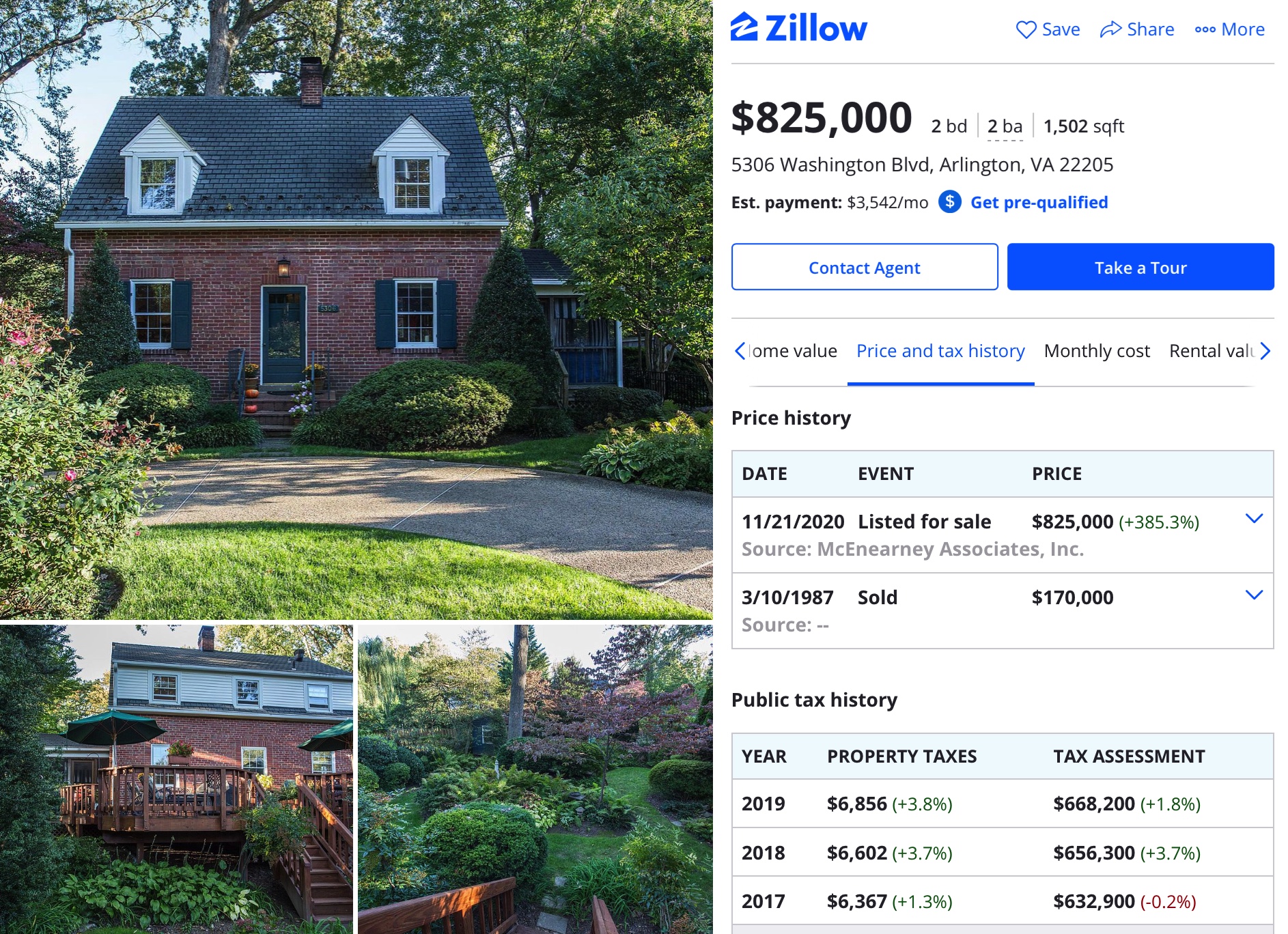
House 2

Can asset pricing make sense of the fact that the much smaller home is worth nearly double the much larger one?

It can.

We can even acknowledge that the economic price of the larger home is much higher, despite location difference. But this does not have to translate into a higher asset price.

The large home, House 1, might have the following characteristics.

Gross rental per year: $40,000
Property tax rate: 3%
Ongoing/upkeep per year: $10,000

The high upkeep costs (aka depreciation) come from its size and features, such as the pool. Notice that property taxes are around $15,000 per year for this dwelling, which is over 3%.

The smaller home might have the following characteristics.

Gross rental per year: $30,000
Property tax rate: 0.8%
Ongoing/upkeep per year: $5,000

Notice the much lower property taxes and upkeep costs. This has a big effect when converting the economic price into an asset price.

I’m going to apply a 2% mortgage interest rate to the asset pricing formula to show what price to expect for these two homes.

House 1
Price = $40,000 - $10,000 / (0.02 + 0.03) = $600,000

House 2
Price = $30,000 - $5,000 /(0.02 + 0.008) = $893,000 

Although the economic price, the rent, is 33% higher for House 1, this doesn’t translate to asset prices. In fact, House 2 is worth nearly 50% more than House 1 under these conditions (my price estimate is a bit on the high side for both as I’m using a round 2% and ignoring any difference in other asset pricing factors). 

Remember, at these prices, each house has the same economic price for buying as it does for renting. House 1 also has an economic price 33% higher than House 2. 

House 1
Interest = $12,000 ($600,000 x 0.02)
Ongoing costs = $10,000
Taxes = $18,000($600,000 x 0.03)
Total = $40,000

House 2
Interest = $17,900 ($893,000 x 0.02)
Ongoing costs = $5,000
Taxes = $7,100($893,000 x 0.008)
Total = $30,000

If you are not looking at housing sale prices through an asset pricing lens, these strange house price differences will only seem to get worse as we enter a super-low interest rate period. Many people will mistakenly attribute the difference in asset price to physical and regulatory factors, like zoning. But if these factors do affect housing, the must do it through their effect on the economic price, the rent, not the asset price.
____________________________
Next post: Why is the share of income spent on housing so stable over time in almost every place?

Why does land have a positive value?

Modern housing economists, following in the footsteps of Ed Glaeser, seem to think that the market price of land should be zero. Land only has a positive value because of artificial scarcity generated by zoning laws.

I am not making this up. This is exactly the theory Glaeser has been pushing for two decades.

According to this view, housing is expensive because of artificial limits on construction created by the regulation of new housing. It argues that there is plenty of land in high-cost areas, and in principle new construction might be able to push the cost of houses down to physical construction costs.
…
this hypothesis implies that land prices are high, not due to some intrinsic scarcity, but because of man-made regulations.

If the price of housing is only the construction cost, that means that the price of the land (location) for housing is zero. It seems a little crazy when you say it this way. Which is why it is usually said in a more sensible sounding “internal logic of my economic model” kind of way. If the input costs to land are zero, and markets are competitive, then the price will converge to input costs. Add in some hedging words, and you begin to sound profound.

But although the model is internally consistent, it is clearly the wrong model. Why?

Land is not a newly-produced good or service, which is the domain of typical economic models of markets, where prices converge to a point that “clears” markets.

Land is instead a perpetual property right to a long-lived scarce asset that can generate income flows. Just like an ownership share of a company, land represents a share of ownership of the finite three-dimensional space.

No one would argue that because there are no input costs for Apple or BHP shares—creating new shares is a legal manoeuvre with essentially no input costs—that competitive trading of these shares will eventually lead to a zero price.

Yet the same argument is thought to be valid when the asset class is land. Maybe if we had public exchanges for land ownership, people would see the commonalities more than they do.

But why can’t land markets be competitive and converge to a zero price? The reason is that the land titles system creates a monopoly over space.

What is puzzling to me is that this monopoly feature of land markets was widely understood issue in the 19th century. It triggered the invention of the Monopoly board game (originally called the Landlord's Game) which demonstrated the monopoly problems inherent to the land titles system and their distributional effect.

Even now, the issue is clear to many who study it. Here’s an entertaining take on the land titles system, and here’s an academic exposé on the issue.

But it remains hidden in the housing supply debate. It is the dog that does not bark in the mystery of rising home prices.

I want to try a new way to communicate the monopoly characteristic of land. The table below shows two ways in which space can be allocated by property titles—either not carved up, with one lot containing all the dwellings on a region, or carved up so that each dwelling sits on its own lot, representing a share of the space. Call it a lot share.

The table also shows two ways in which a property title (a lot or lot share) can be owned—either by a single person or by many people. 

If we have one giant lot that contains all the dwellings in a region, and that lot is owned by a single person, then that clearly makes the land market a monopoly. This is a situation similar to company towns, where a company builds housing on land near a natural resource or mine to provide local accommodation for its workers. 

Even if that single large lot was owned by many people, such as through a corporate structure, a co-op, or another legal mechanism, it would still be seen as a monopoly. 

Although many people own a share of the total space, the space is only one land lot, one property title, so the number of owners does not matter. All the owners’ interests are the same. The monopoly outcome is expected. Even if there are 100 dwellings, with each occupant owning a one-one-hundredth share in the company, it is still a monopoly. 

Now let’s carve up ownership a different way. Rather than owning a one-one-hundredth share of a company that owns the single lot containing all one-hundred dwellings, each household ones a one-one-hundredth share of the lot. Rather than subdivide the company structure, the lot structure is subdivided into lot shares.

Each household still owns a one-one-hundredth share of the space. 

Does this change to the legal structure of ownership change the economic outcome? If so, why?

In Ed Glaeser’s model world, switching the ownership structure of this area so that each household went from part-owner of the entity that owns the space to part-owner of the space would immediately crash the price of land to zero.

This is internally consistent with his model—land has a positive value only because of “artificial” monopoly features of the market. Once you “create competition” between landowners, prices fall to input costs, which are zero. 

One detail that many people overlook when they make the “many owners = competition” assumption is that it is simple for even large numbers of people to converge to the monopoly outcome. Trial and error gets you there. In fact, it is not clear that there is a mechanism that gets a market like land away from the monopoly price. Who would deviate?

This is why, for thousands of years, property titles systems have been stores of wealth—an asset traded in markets and subject to cycles like any other asset class. Thinking about land as an asset explains housing price patterns observed much more than any plausible supply-side story. But unfortunately, admitting that the land market is a monopoly is problematic for economists as it undermines many theories (especially involving anti-trust). It also demonstrates that much of our macro-economic policy—monetary policy, taxation policy, and banking policy— is having large effects on housing markets.

If a politician says they want affordable homes, they are lying

First published at Sydney Business Insights.

What chance does a political party promising to radically reduce home prices to improve affordability have of getting elected?

The electoral calculus is simple. There’s no chance. If they say they want to, they are lying. This reality is why the Federal Budget included policies to boost housing demand but do not expand the supply low-cost housing alternatives.

The reality is that the richest seven out of ten households own $7 trillion of housing wealth in Australia. That’s $7,000,000,000,000, or seven million-million dollars. Every single 1% price decline wipes off $70 billion from the balance sheets of these households.

Yet we find ourselves in the bizarre situation where politicians are obliged to talk like they want home prices to be lower and “more affordable” but must act in ways that support higher home prices. We can only conclude that our housing market is exactly where we Aussies want it.

Consider these figures from a recent working paper I produced with Josh Ryan-Collins. Bank lending for housing grew from 20% of GDP in 1990 to 80% of GDP today. Business lending for investment grew from 35% to 40%. We have used the power of banking to bid up house prices by trading them with each other while neglecting investment across the business community. No one saw a problem with this because it helped house prices grow.

While the media celebrated the ‘booming’ real estate market, during the 2010s homeownership rates fell from 70% to 65% – back to levels last seen in the 1950s. The main difference between now and then is that governments of the 1950s intervened strongly to get more people into homeownership with public loans for new homes, direct public land provision, and rent controls on landlords that prompted many to sell to owner-occupiers. The state also provided extensive public housing alternatives, with nearly 15% of new supply being public housing in the 1950s compared to just 2% across the past decade.

You can see why our housing situation is so attractive for the homeowning cohort by comparing the economic returns from housing to wages. In the seven years to June 2019, the median Sydney home earned as much from rent and capital gain as the median Sydney full-time worker! This is astonishing. Capital growth of all land, not just residential, went from 9% of GDP on average between 1960 and 1980, to 17% of GDP since 2000, roughly twice the relative return to landowners than just 40 years ago.

Reversing these trends will benefit younger generations who are resigning themselves to the fact that homeownership is beyond their reach. While the “bank of Mum and Dad” has helped shift some housing wealth down the generations, it has entrenched inequalities. Waiting for inheritances won’t work either. The average age of receiving an inheritance is 64 years.

Housing is a defining social issue of our time. We have many technical solutions in our working paper, but the sheer economic value at stake and the political realities of this mean that change won’t be easy.

The problem with making home ownership more affordable is not a small elite locking the majority out: a lot of us (ie homeowners) have a lot to lose if we want to share the joy of a home of one’s own.

Low interest rates will boost home prices, as designed

Interest rates are the main story for home prices in Australia. With our deregulated banking system, all demand for mortgages can be satisfied (conditional on servicing criteria). With an active and tax-advantaged investor market, this only adds to the tendency for the housing market to converge to the asset-pricing equilibrium, where mortgage interest on the home price substitutes equally for rent, along with some adjustment for ownership costs and expected capital gains. 

Here’s the basic gist of the interest rate effect for a typical home that saw rising nominal rents until recently, but where rents are expected to see no nominal growth over the next decade.

Despite rents in this example rising only 47% in nominal terms over four decades, the equilibrium asset price (where mortgage interest substitutes for rent) increased by 338%. 

Notice that the 2% point decline in interest rates has a larger effect when interest rates are lower. Halving interest rates should double prices, all else equal (and vice-versa). But that requires only a 2.5% point drop from 5%, but a 4.5% drop from 9%. 

The latest bout of monetary policy that took mortgage interest rates from around 4.5% to 2.5% is nearly a halving, which implies scope for a near doubling of prices. Even if Sydney and Melbourne prices were far above the equilibrium due to a recent speculative period, this interest rate decline will bail out speculators and support those higher prices. 

This is why I see mostly upside for home prices in Brisbane, Adelaide, and even Perth in the next few years. Gross yields for Sydney and Melbourne houses are around 2.6%. But they are 3.8% in Brisbane, 4.2% in Adelaide, and 3.8% in Perth. A 0.5% point decline in yields in Brisbane, would, for example, see a 15% price gain. 

If you can borrow at 2.5% the maths looks like this for a home currently rented for about $400/wk ($20,000/yr).

Annual rent - $20,000
Price at 3.8% gross yield - $526,000
Interest on price (2.5%) - $13,150
Interest on price (2%) - $10,500

If you buy with a 2.5% mortgage instead of renting, you get nearly $7,000 year in your pocket to cover ownership costs and repay the mortgage. If you can get a mortgage interest rate closer to 2%, that gives you nearly $10,000 per year to cover these costs. 

Even if you expect rents to fall 10%, this doesn't change the asset-pricing arithmetic much at all. 

If you don’t expect prices to fall rapidly, buying makes good financial sense with low interest rates and high housing yields.

UPDATE: It is now cheaper to pay the interest on a mortgage than pay the rent in the major capitals on average (see blue line dipping below one). The below image is that ratio of the interest rate to the gross yield. It also shows the repayment for a 30-year mortgage as a ratio of the rent in orange. 

Superannuation fees to rocket

These comments were reported in The Australian on 10 Sept 2020.

_____________________________

At the current 9.5% compulsory contribution rate, Australia’s superannuation system is already an unbelievably expensive retirement income system. It employs 55,000 people and costs $34 billion in fees each year to deliver only $40 billion in retirement incomes (see the Scrap Superannuation report here). Increasing the compulsory super contributions rate to 12% of wages will do little to support retirement incomes while adding substantially to the economic cost of the superannuation system.

“The economic cost of super to members comes in the form of direct fees, which are around 1% of super balances on average, as well as the foregone investment returns from those fees,” says Dr Cameron Murray, economist and research fellow at The University of Sydney.

“For a typical earner with a 40-year work-life they can expect to have a real super balance of $743,000 at retirement, having paid about $108,000 in fees over their lifetime,” said Dr Murray [1].

“But those fees could have earnt an additional $74,000 in investment income, meaning the total economic loss is $182,000 over a lifetime.”

“Raising the compulsory super contribution rate to 12% will see funds charge this typical earner an extra $28,000 in fees over their lifetime, losing an addition $20,000 of investment income” concluded Dr Murray. 

“Even if funds improve their performance and fees fall by half, the compulsory rate increase will see this typical worker lose an extra $16,000 to fees over their working life, and around $10,000 of investment income.”

“The super system is one of the most economically inefficient ways to support retirement incomes. Raising compulsory contributions will only add to these costs, creating even more jobs for blow-hard spreadsheet monkeys who pay themselves from our retirement savings.”

______________________________

[1] First ten years of work-life at 70% of average full-time wage, and last ten years at 30% above the average full-time wage, with middle twenty years at the $90,000 average full-time wage. All returns and costs are in real terms. See Table 1 and 2 for a result summary.